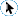First Automatic Totalisator: More Details of Horse Adders

The central shaft has two differentials and two driving chains, one to the 12-shaft and one to the 17-shaft. The central shaft is really a small adder that combines the rotations of the 12-shaft and 17-shaft. The details are a bit difficult, but the following diagram, viewing the central shaft from above, might help explain what is happening.

In the diagram the directions of rotation at the top are given by the arrows. The central shaft rotates counter-clockwise. It is pinned to the back planetary gear that also rotates counter-clockwise (viewed from the front of the machine). The first planetary gear may rotate if the second planetary gear also rotates counter-clockwise or the front end cog rotates clockwise. The front-end cog drives the 17-shaft. The difference in sprocket-wheel diameter of the central shaft and the 17-shaft means that a 1/20 rotation of the 17-shaft is represented as a 1/10 rotation of the central shaft front-end cog, but the rotation passed through to the back-end planetary gear is halved. So a 1/20 clockwise rotation of the 17-shaft becomes a 1/20 counter-clockwise rotation of the central shaft. The rotation of the 12-shaft is similarly doubled by the gearing. However, the 12-shaft drives the front planetary gear and in this case the rotation is not halved. So a 1/20 counter-clockwise rotation of the 12-shaft becomes a 1/10 counter-clockwise rotation of the central shaft and so represents a value twice that of the 17-shaft, so we can deduce that the 12-shaft is for 20 shilling bets and the 17-shaft for 10 shilling bets.

Support for our interpretation of the horse adders comes from the 1915 patent application. This introduces the idea of having differing numbers of teeth on the escapement wheels to represent different sized bets (20 teeth for 10/-, 10 for 20/-, 4 for 5 pounds etc.) The patent says:

The construction by which it is made practicable to compute by means of an epicyclic train on one shaft a plurality of dissimilar values is one of the the leading features of the present invention, which distinguishes it from known apparatus of its class in which the several different orders of values must be separately computed on separate trains of epicyclic gearing, and the movements of these several trains compounded together in another epicyclic train upon a collecting shaft, through which motion is transmitted to the indicator.

In later machines it was still common to have multiple shafts but this seems to have been because smaller shafts were more reliable and practical.

How the 5-pound bets were counted is unknown. There does not appear to be an escapement wheel with fewer teeth so the 5-pound bets must be counted in some ad-hoc manner. It remains a puzzle but could be something to do with the central shaft (allowing the back-end cog, assumed fixed, to actually complete one full rotation would have the right result).